Torsional Deformations of a Circular Bar

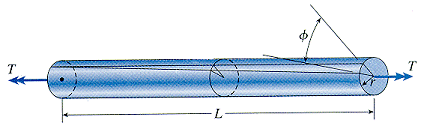
pure torsion

where every cross section of the bar is identical and subjected to the same internal torque

• Due to symmetry, all cross sections remain plane and circular and all radii remain straight.
• The bar is also said to be in pure shear.

 

angle of twist, φ

the angle through which one point on the bar has been twisted with respect to another point

• If every cross section has the same radius and torque, then φ will vary linearly along the bar.
• If φ is small, then the length and radius of the bar will not change.

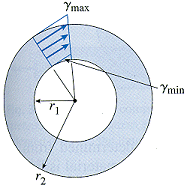
angle of twist per unit length (rate of twist), θ θ = φ / L   shear strains, γ γ = cθ where c is the radial distance from the center to the point under consideration. Note that shear strain is a minimum at the inner most surface (zero at the center) and a maximum at the outer surface.