In general, predicting the state of stress and strain on the surface of a torsion member is very difficult. Fortunately, the special case of circular torsion members (either solid or hollow as long as there is axial symmetry) is relatively simple. Elementary theory predicts a state of pure shear stress on the surface of a circular torsion member. Pure shear is defined as a state of shear stress in the absence of normal stresses.
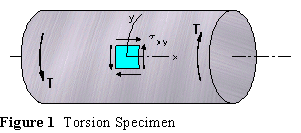
On the surface of a circular torsion member (Figure 1) the state of shear stress (in the axial and circumferential directions) is given by:
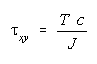
where T is the applied torque, c is the radius and J is the polar moment of the cross-sectional area. For a solid circular member:
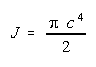
Although the shear stress equation above contains no elastic constants, review of the derivation will show that linear elastic theory was assumed.
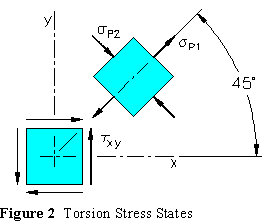
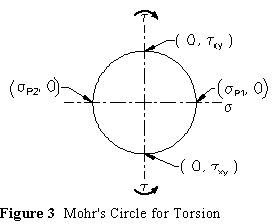
It is important to realize that pure shear stress can exist only for one orientation of the coordinate frame. For all other orientations normal stresses will exist, and at ±45o to the pure shear axes we find principal stresses (and thus no shear stress). It is easy to show with Mohr's circle (Figure 3) that the principal stresses are equal tensile and compressive stresses with the same magnitude as the torsional shear stress. The conclusion is that the state of pure shear stress is equivalent to a biaxial state of equal tensile and compressive stresses oriented at 45o to the pure shear axes (Figure 2).
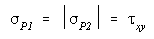
The surface shear strain associated with the axial-circumferential directions is given by:

where q is the relative angle of twist (in radians) over the length L. This relationship holds not only for the linear elastic range, but also for inelastic torsion. We can measure the angle of twist with a device called a troptometer. Since the angle of twist is directly proportional to shear strain, we are in effect measuring shear strain.
Some troptometers measure angle of twist in either radians or degrees. Our troptometer will allow us to measure arc length in inches. If the arc length is divided by the radius of the troptometer (from the center of the specimen to the arc length scale) the result will be the relative angle of twist in radians for our gage length.
Although we can calculate the modulus of rigidity G from values of the modulus of elasticity E and Poisson's ratio
determined in a tensile test, it is sometimes desirable to obtain it directly. We can use the torsion test for the direct determination of the modulus of rigidity from the relationship:

or alternately
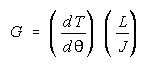
The latter formulation allows us to determine G from the slope of the torque vs angle of twist curve.
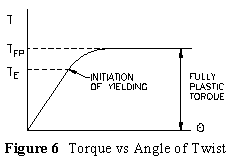
Care must be exercised in determining the slope of the T vs q (or t vs g) curve (Figure 6) since the deviation from linearity is very gradual. In our discussion of inelastic torsion we shall see how we can determine the theoretical limit of linear action in terms of the fully plastic torque (TFP).
We shall assume we have a material which exhibits linear elastic, perfectly plastic behavior. This is a good model for the behavior of carbon steel which exhibits a yield point. The shear stress vs shear strain curve for such a material would look like the axial stress vs strain curve (Figure 4). If the strain is increased a sufficient amount after yielding is initiated the stress will again increase, just as it did in the tensile test.

The process of yielding in a torsion member is more complex than in the tensile member. In the tensile member, the entire cross-section was subjected to a constant stress and thus yielding occurred simultaneously across the entire section. In the torsion member yielding starts at the outside and progresses inward with increasing torque (Figure 5). As the torque increases we finally reach a fully plastic condition where the strain (and thus angle of twist) is increasing while the torque (TFP) is approximately constant. At the fully plastic torque the entire cross section is yielding. The resulting torque vs angle of twist curve is shown in Figure 6.

There are problems with this theoretical state of fully plastic torsion because it calls for a discontinuity of stress at the center of the specimen. In spite of this defect in our model, we find we can in fact approach a fully plastic torque. At the fully plastic condition the torque will remain essentially constant while the angular deformation increases. If we continue to twist the specimen the torque will again begin to increase. The increasing torque which follows the plastic action is directly related to the increased stress which follows yielding in the uniaxial loading of carbon steel (i.e. after sufficient yielding at essentially constant stress, the stress again increases with additional deformation).
It is easy to show that for the fully plastic state the relationship between torque and shearing yield point stress is given by:
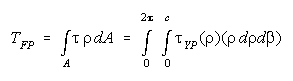
Thus
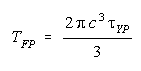
If we plan to determine the modulus of rigidity G, we need to know the theoretical limit of linear action. We can determine the torque required to push us to the limit of linear action TE by solving for the torque required to make the shear stress at the surface just reach the shearing yield point stress tYP. Utilizing the linear elastic torsional stress equation (t = Tc/J) we obtain:
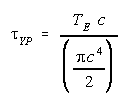
or
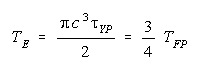
For purposes of determining the modulus of rigidity, one should work with data which does not exceed seventy-five percent of the fully plastic torque. For materials which do not exhibit a yield point, stay well below the apparent limit of linear action.
Mechanics of Materials, by Higdon et al., 4th Ed., Wiley, 1985, pp 179-185, 196-199, 204-205.
Mechanics of Materials, by Hibbeler, Macmillan, 1991, pp 171-182, pp 192-195, pp 232-236.
Mechanics of Materials, by Hibbeler, Macmillan, 2nd Ed, 1994, pp 177-188, pp 198-202, pp 238-244.